Can you recall feeling frustrated when a teacher professional development opportunity you were a part of seemed disconnected from the day-to-day realities of your classroom? Our schedules are so full as teachers, it can be hard to justify sitting through a training that doesn’t have immediate applicability to what you’re teaching (and the questions you have about what you’re teaching) right now!
We had this in mind when we began to plan for a deep dive into the grades 3-5 fraction progression with Newport-Mesa Unified School District teachers. As we planned our training, we had a couple of key questions:
- What is the best structure for content-embedded professional learning?
- How do we balance a desire to help teachers dig deeply into fraction concepts while staying focused on instructional practices and student learning?
- What are the best ways to specifically learn about planning for instruction, facilitating math learning for students with different learning needs, strategies for noticing and responding to student thinking, and developing deep understanding of critical fractions concepts?
Research on Professional Learning Structures
To determine the best structure, we first had a look at the research. The report Professional Learning in the Learning Profession: A Status Report on Teacher Development in the United States and Abroad, examined what research has revealed about professional learning that improves teachers’ practice and student learning. According to the report, “Research suggests that professional development is most effective when it addresses the concrete, everyday challenges involved in teaching and learning specific academic subject matter, rather than focusing on abstract educational principles or teaching methods taken out of context.” Additionally, “teachers themselves judge professional development to be most valuable when it provides opportunities to do “hands-on” work that builds their knowledge of academic content and how to teach it to their students.”
A Model that Balances Content Learning and Hands-On Practice
Out of this research, the concept for Fraction Lab was born. We planned to facilitate content learning sessions for teachers, including time to think about planning for instruction. We then planned to bring kids in and give teachers the opportunity to try out math tasks with students and practice noticing and responding to student thinking—this would be immediate hands-on practice to reinforce the learning and help the teachers see where they still might have questions.
We asked students to attend Fraction Camp for half-days during a week of the summer. Teachers at the host school selected students going into grades 4 and 5 who would benefit from additional work with fraction concepts. To prepare for the work we would do with students, we needed to get a sense of the knowledge and understandings students were bringing with them. We looked at the fraction understandings developed within grades 3 and 4 and designed assessment tasks that would allow us to gain insight into student thinking. We administered the paper-pencil task and one interview question to students. This allowed us to narrow in our focus on fractions to particular fraction concepts that would benefit the students the most, and build on what students knew and understood.
While our model was specific to fraction concepts in grades 3-5, this model of content-embedded, job-embedded professional learning could be adapted for any math concept. Additionally, our Fraction Lab was run during the summer over seven full days; however, the timing and structure could be adapted to meet the schedule configuration of a PLC or school-based professional learning sequence during the school year.
The Fraction Lab consisted of four phases:
- Content learning
- Planning for teaching
- Teaching
- Reflection
Phases 2 through 4 repeated in cycles for five days. We’ll break down each phase and share the resources we used.
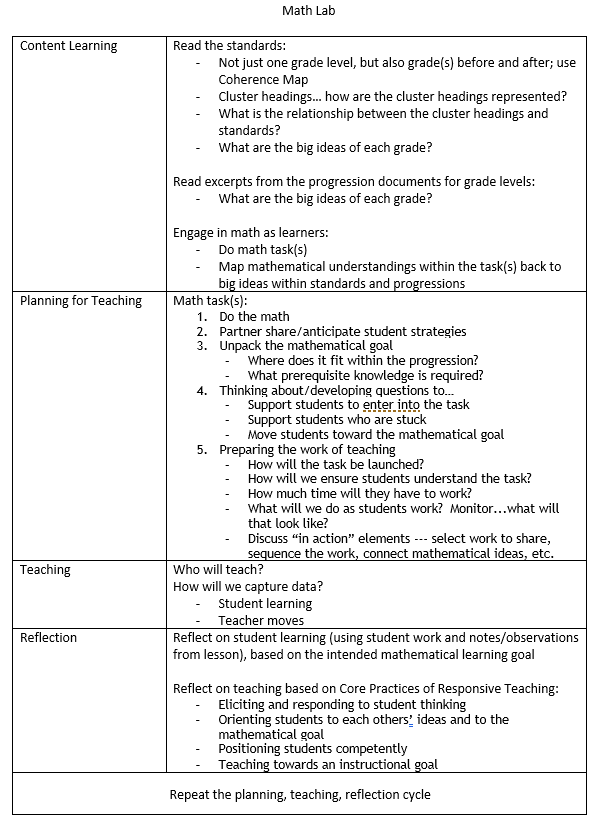
Content Learning
Educational Researcher Deborah Ball says, “Teachers must know the subject they teach. Indeed, there may be nothing more foundational to teacher competency” (Ball, et al., 2008).
So that’s where we started—with learning to help teachers really “know” fractions. We launched two full days of a deep dive into the state standards and Progressions. This consisted of:
- Reading the standards and thinking about the big ideas of each grade level in 3-5 fractions.
- Reading excerpts from the 3-5 NF Progression Document and pulling out the big ideas of the grade levels.
- Engaging in mathematics as learners: completing fraction math tasks from grades 3 to 5, with discussion on the mathematical concepts within the tasks, mapping back to the standards and Progressions.
Planning for Teaching
On the afternoon of the second full day with the teachers, we shifted to begin planning for teaching the students who were going to be in the classroom the following week. We had given the students a pre-assessment and we started by unpacking the data and making connections to the standards and the big ideas of grades 3-5. We looked at the data to identify the critical fractions concepts the students needed to spend time with.
Based on student understandings and the fraction progression within the standards, we decided to focus on the understanding of fractions as numbers, understanding and naming the whole, equivalence, and composing, decomposing, and comparing fractions. With these concepts in mind, we identified two instructional routines that would be part of our daily work: choral counting and a yarn number line. We decided to incorporate these two routines for the learning affordances they provided for students and teachers. For students, these routines would allow them to think about fractions as numbers as they counted by fractions, considered magnitude and number relationships, and looked for and made use of structure within the number system.
From a teacher learning lens, these routines would provide opportunities to work on Four Core Practices of Responsive Teaching: Eliciting and responding to student thinking; Orienting students to each others’ ideas and to the mathematical goal; Positioning students competently; and Teaching toward an instructional goal.

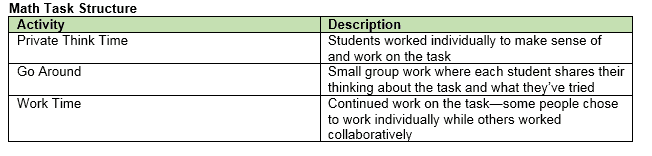
We then designed a planning protocol that began with teachers doing the math tasks. This provided an opportunity to think about the math that students would encounter, consider prerequisite knowledge that would be needed to access the task, and anticipate student strategies and possible misconceptions. The teachers did the math tasks in the exact same structure that we would later replicate for students.
This model was selected to ensure that everyone had time to process and think about the task and then an opportunity to hear the perspectives of others and work collaboratively. Engaging in this process with the teachers was important not only in unpacking and making sense of the mathematics content and student thinking around it, but also to think about how situating the task in this way could provide access and opportunities for students with different learning needs and styles.
After doing the math, the teachers unpacked the learning goal of the task together, discussing key understandings and disciplinary ideas. We discussed where these understandings fit within the progression of learning as well as prerequisite knowledge being built upon. This supported teachers in seeing coherence within and across the standards and Progressions.
The next step was thinking about and developing questions that would be used by teachers within their instruction. We designed questions that did the following things:
- Supported students in entering the task
- Supported students who were stuck
- Made student thinking visible
- Moved students toward the mathematical goals.
The final element of planning was preparing the work of teaching. We broke our thinking down in the following way, building on the foundations of 5 Practices for Orchestrating Productive Mathematics Discussion.
Launching of the task:
- How will the task be launched?
- How will we ensure students understand the task?
- How much time will they have to work?
Our role as teachers:
- What will we do as students work?
- How will we monitor…what will that look like?
- How will we keep track of what we hear?
- What type of things are we looking for and how will we record what we notice?
“In-action” elements:
- How would we would use student work to make the mathematics explicit for all students?
- Which solution strategies would we look for to share?
- How we would sequence the solution strategies, and how we would make connections between strategies?
We picked different teachers from the group to co-teach each task and teachers had an opportunity to rehearse their teaching.
Teaching
While some of the teachers in the Fraction Lab taught to the students each morning, the others of us observed, took notes, and interacted with students, asking the questions we had planned. We also called Teacher Time-Outs if we felt stuck as instructors. This allowed us to pause the lesson, collaborate on a particular instance of teaching, gain input from the other teachers in the room, and then enact the suggestions.
The students appreciated the learning environment too, where even the teachers had learning to do and sometimes needed help! Some of our participants also video recorded the lessons and the student groups working, so that we could analyze the teaching and the student thinking based on the video evidence. We also collected the student work from each task to use for analysis.
As a result of the lab model and the number of teachers in the room at all times, we were able to get insight into the thinking of every student with every task. While we realize this doesn’t mimic the daily work of teaching, it does afford the opportunity to consider the type and depth of information that’s useful. Having multiple perspectives is also helpful in thinking about noticing, interpreting, and responding to student thinking.
Reflection
Each day, after the students went home, we spent time on reflection of the teaching practice as well as the student thinking. We looked over the student work and thought about it in light of the mathematical learning goal for each task. We also referred to our notes and video recording of students talking to discuss their level of understanding of the fraction concepts. We then looked over our plan and made adjustments if necessary, based on the student thinking.
Additionally, we reflected on the teaching from the day based on the Four Core Practices of Responsive Teaching. The teacher-observers again referred to their notes and the videos from the day to reflect and identify areas of strength and areas for improvement for the next day.
This deep content-embedded professional learning was transformative for the teachers and the students. Situating teacher learning in the context of what they do every day (teaching!) is a model of professional learning that could be adopted by grade-level/content teams, PLCs, and school-based communities. A teacher participant said it best: “It was crucial to have the students as part of this training so that we could see how they solved the problems. I think what we learned from the students will stick with us so much more than if it had just been a lecture on what concepts students struggle with in fractions.”



















Thank Jody and Jennie. I appreciate you sharing your design for integrating work on both content knowledge and teaching practice simultaneously. This in my own experience has been a powerful approach for teachers over the past few year after learning about this approach from the LTP Project. High-leverage math instructional activities such as Choral Counting, Number Talks, and the use of problem solving (equal sharing, multiple groups) make this process more integrated. For those who read this blog post some additional resources can be found with resources at these two websites: https://tedd.org/ & https://www.stenhouse.com/content/choral-counting-counting-collections & https://www.stenhouse.com/content/choral-counting-tool?destination=node/3261